We need to talk about how we talk about swing... Part 1 - Degrees of Swing
In the modern world of cricket data, swing bowling is left in the dark ages.
For this first post, I thought I would write about my old nemesis, the Degree of Swing. Anyone who has watched test match coverage in the last few years, will no doubt have seen swing measured using an ‘average degree of swing’, either for a session or a team. This statistic is used to quantify how much a ball is moving through the air, and measures the change in angle of the ball direction from its release to when it bounces.

The tweet above shows an example from the excellent CricViz analytics team, comparing the swing achieved by the Australian swing bowlers in two innings on two different days. However, the issue I have with this information, is that it doesn’t actually tell you anything about how the ball is swinging, why the result has occurred, or what is likely to happen in the future.
Now obviously there is not a lot of room for explaining the nuance of swing bowling in 280 characters, so let’s focus on the simplest element of measuring swing: how the ball moved through the air. The aim of this post is to show you that there are better ways of measuring swing that provide you with more information, if you dig a little deeper into how swing bowling works. Now be warned, there will be references to geometry and algebra, but even if GCSE Maths features in your worst nightmare, I promise it’s worth the ride.
“Pitch it up, give the ball a chance to swing!” - Anyone who has ever played, coached, or commentated on cricket
The tweet at the start of this post highlights a key aspect of measuring swing: the amount a ball swings is intrinsically linked to the length of the delivery. This fact is known to any player who has watched a floaty, full delivery leave the bowler’s hand and instinctively pictured a glorious cover drive in their head, only to find the ball leave them in the air, find the edge, and nestle in the wicket keeper’s gloves. As well as this, the ball curves through the air, moving further sideways the closer it gets to the batter, as seen in trajectories from ball-tracking measurements.

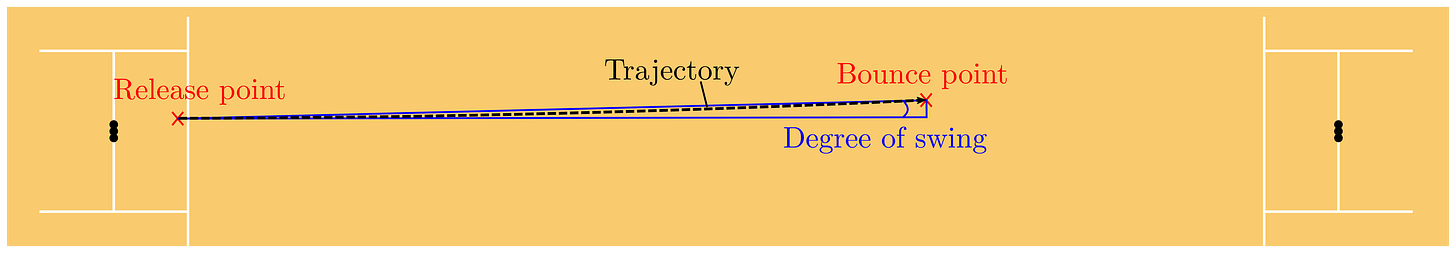
Before we dissect why this is the case, let’s consider how well the degree of swing copes with these key properties of swing. For that, we need to draw a picture of a swinging delivery from a viewpoint directly above the pitch.
This diagram shows the data points recorded from ball-tracking systems which are used to measure the degree of swing in professional games. The red crosses show where the ball is released and where it bounces, and the dashed black line shows the path the ball takes to get there. A measurement of ‘degrees’ is used to measure the angle between two straight lines, and so a triangle is drawn between the release and bounce points to calculate the degrees of swing value.
Now you may have spotted an issue with the diagram above (other than the fact that the delivery is a wide half-volley). The triangle used to measure the degree of swing does not track the trajectory of the delivery, as it uses a straight line to follow a curve (more specifically a parabola, but we shall come to that).
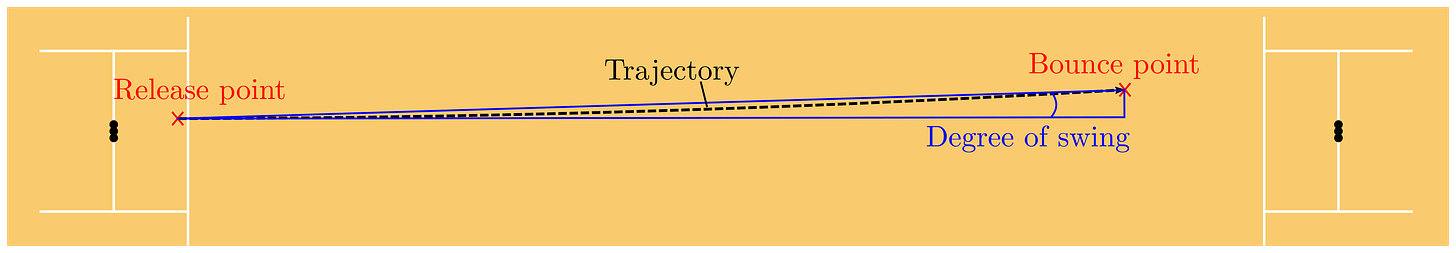
The second diagram shows the exact same trajectory shape for a shorter delivery which pitches further from the batter. The degree of swing measurement now provides a different value for the same trajectory, simply because the delivery length is different. Granted, the distance that the ball has swung, shown as the vertical blue line below the bounce point, is also different, but as we know, the fuller the ball, the more it swings, so we are not gaining any new information.
Practically, this means that you can achieve the same degree of swing by either:
Bowling a full ball that does not swing very far
Bowling a length ball that swings a lot
Therefore, the degree of swing does not tell us how much a bowler can swing a ball, unless their delivery length is also known.
Having a metric that relies on another measurement for context is clunky and inefficient, and means that two scenarios cannot be easily compared through one number. On top of this, the measurement itself is not even particularly intuitive. Can anyone tell me what one degree of swing looks like? Or how different that is to 1.3 degrees? I understand that people have a feeling for different angles, but when you can’t visualise the difference between two values, the unit becomes meaningless.
So, after all the ranting about how the current measure of swing isn’t very good, it’s time to address the elephant in the room: is there a better way to measure swing? To answer that, it’s time to get our first taste of the physics of swing bowling.
I have split this topic into two parts, and will either publish a second article looking at aerodynamic measures of swing, or I will update this one once I have time. Either way, stay tuned for the next update!



Thank you for writing this post! It expresses something I’ve thought about for a while which is that the amount of swing understood as the amount of curviness of a trajectory can’t be described merely by release point, pitch point, and interception point data. The same three points can many different curves intersecting them. I think people maybe started using degrees for spin bowling and seaming deliveries and just decided to use it for swinging deliveries as well.